Premium content
Access to this content requires a subscription. You must be a premium user to view this content.

poster
Approximation of microwave assisted magnetization switching field applied with incident angle
Microwave-assisted magnetic recording (MAMR) 1 has attracted much attention as one of the recording techniques for the ultra-high-density recording. Theoretical analysis of microwave-assisted magnetization switching (MAS) was studied in the case when DC field was applied parallel to the anisotropy direction 2, 3, which indicated that the effect of microwave assistance was explained by the equivalent magnetic field applied along anisotropy axis given by ω/γHk. However, the analysis of MAS is quite complex when DC field is applied non-parallel to the anisotropy axis. This study proposes an approximated estimation method when DC field is applied non-parallel to the anisotropy axis.
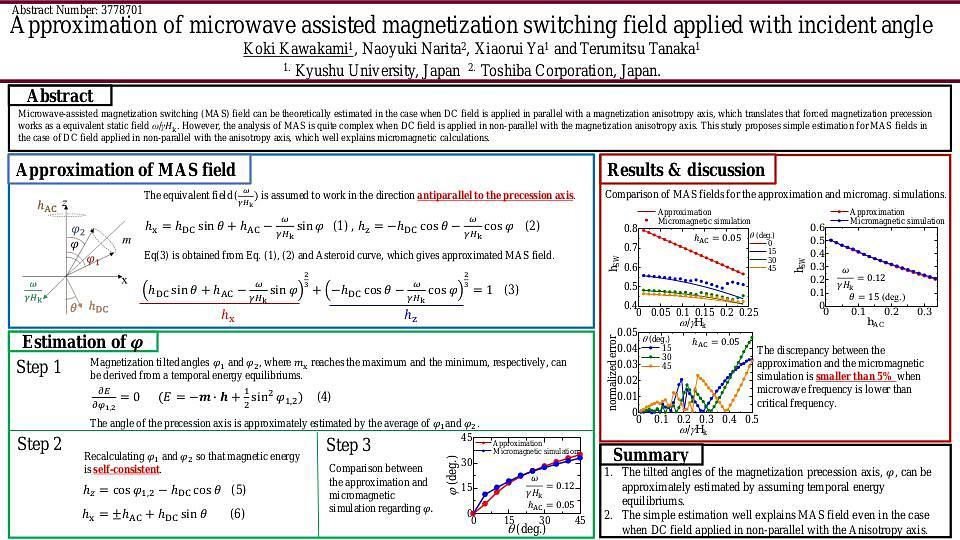
Fig. 1 shows the schematic drawing of the normalized equivalent field, ω/γHk due to magnetization dynamics. Normalized DC field (hDC) is applied at θ with respect to the anisotropy axis. Circular polarized normalized field (hAC) is applied in the x-y plane. Here, the angle of the precession axis φ is approximated by the average of φ1 and φ2 which stand for the angles between the anisotropy axis and the magnetization directions at the maximum mx and the minimum mx, respectively. The direction of the equivalent field is defined to be anti-parallel to the precession axis in this study. In our previous study, we confirmed the in-plane and z components of the normalized effective fields were approximated by Eqs. (1). These equations well explained micromagnetic calculation and the resultant switching fields (hSW) well agreed with the asteroid curve Eq. (2).
hxy =hDCsinθ - ω/γHksinφ +hAC , hxy =hDCcosθ - ω/γHkcosφ Eqs. (1)
hxy2/3 + hz2/3 = 1 Eq. (2)
Hence, hSW is given by φ. φ1 and φ2 are obtained from the equilibrium condition (∂E/∂φ1,2 =0) of the magnetic energy defined by Zeeman and anisotropy energy. Fig. 2 compares the approximation and micromagnetic calculation for various θ when hAC is 0.05. hSW are plotted as a function of the microwave frequency lower than critical frequency. The error between the approximation and micromagnetic simulation is less than 2% which is quite small.
References:
1 Jian-Gang Zhu, et al., IEEE Trans. Magn., Vol. 44, pp.125-131 (2008).
2 G. Bertotti, et al., Phys. Rev. Lett., Vol. 86-4, pp. 724-727 (2001).
3 S. Okamoto, et al., J. Appl. Phys., Vol. 107, 123914 (2010).

Fig. 1 Schematic illustrations for the equivalent field.

Fig. 2 Comparison between approximation and micromagnetic simulations.