Premium content
Access to this content requires a subscription. You must be a premium user to view this content.

poster
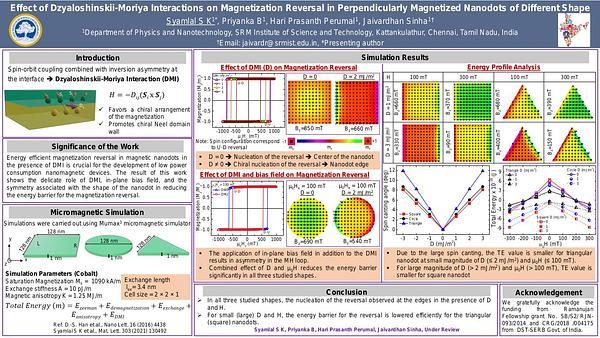
Magnetization reversal dynamics of a triangular shaped magnetic fractals
Fractals are composed of self-similar structures across different length scales, which look similar under different magnifications. They possess a non-integer dimension with a self-similarity and scale invariance, which is commonly referred to as the Hausdorff dimension or fractal dimension. Romanesque cauliflower, fern, frost, and snowflakes are popular examples of naturally observed fractals. The concept of fractals namely, Sierpinski square and triangles are also implemented in magnetic systems to study the modification of spinwave dynamics 1, 2. It is also important to study their magnetization reversal process for their further use in spintronics. Here we have investigated the magnetization reversal process of a Sierpinski triangle which is a nonperiodic but ordered fractal structure.
To understand the magnetization reversal process of Sierpinski triangles with the increase of the iteration level of the fractal structure are studied using a micromagnetic simulator namely object oriented micromagnetic framework (OOMMF) 3. The magnetic parameters used for the simulation are taken from Ref. 1. The hysteresis loop (M-H curve) is calculated for a structure going from simple geometric structures toward a Sierpinski triangle. TRI-0 is an equilateral triangle with the side length of 3 μm. To generate TRI-1, an equilateral triangle is removed from the center of TRI-0. To further generate, TRI-2 and TRI-3 an iteration process with a scaling factor of ½ is used. Due to the structurization, the coercivity and saturation magnetization are increased significantly with the increase of the iteration number as shown in Fig. 1(a). The simulated magnetization images during the reversal for Tri-0 and Tri-4 are shown in Fig. 1 (b) and (c) for three different magnetic fields. The interesting thing to note is that magnetization reversal occurs by the creation of a vortex. The observations have a great impact on their applications in spintronic and magnonic devices.
References
1. J. Zhou, M. Zelent, Z. Luo, V. Scagnoli, M. Krawczyk, L. J. Heyderman, S. Saha, Phys. Rev. B105, 174415 (2022)
2. C. Swoboda, M. Martens, and G. Meier, Phys. Rev. B 91, 064416 (2015).
3. M. Donahue and D. G. Porter, “OOMMF User’s guide, Version 1.0,” NIST Interagency Report No. 6376 National Institute of Standard and Technology, Gaithersburg, MD, 1999,
URL: http://math.nist.gov/oommf.

Fig. 1 (a) Simulated hysteresis loops for different samples. Simulated magnetization images at various applied magnetic field values marked by ‘1’, ‘2’ and ‘3’ for (b) Tri-0 (c) Tri-4 a single triangle is taken from the whole structure