I.Introduction
Understanding of excess loss in electrical steel sheets, which is the difference between the measured iron loss and the sum of static hysteresis loss and classical eddy current loss 1, is difficult as it is related to non-repeatable dynamic domain wall motion inside the steel 2, whereas it is normally agreed upon that the anomalous eddy current loss, which is due to the micro eddy current generated by domain wall motion, is one main source of the excess loss for grain-oriented steel sheets 3. One famous model coping with the anomalous eddy current loss is the Pry and Bean domain model 4 and we have established a numerical domain model based on it 5. However, this model cannot model the skin effect of the flux density and it cannot represent the nonlinear BH curve of the steel sheets. In this paper, a simple numerical method using multiple boundary conditions is proposed to calculate the micro eddy currents and the classical eddy currents simultaneously while considering the nonlinear B-H constitutive relation of steel sheets.
II.Analysis model and Conditions
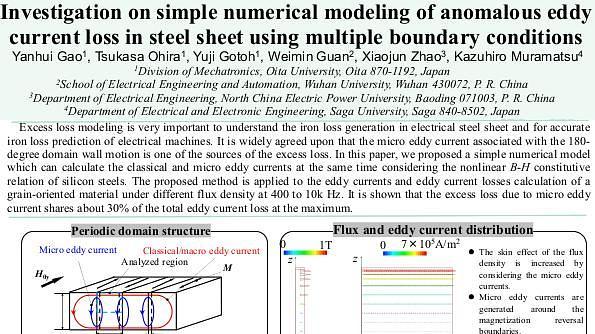
The domain pattern of grain-oriented steel sheet is considered as a simple periodic one as shown in Fig. 1. Half of one periodic region is chosen as the analyzed model. Micro eddy currents are generated around the domain walls when the domain wall moves. In the proposed analysis, the overall alternating magnetization to induce the classical eddy currents is imposed by using one Dirichlet boundary condition. And the local magnetization change due to domain wall motion is imposed by using another Dirichlet boundary condition to induce the micro eddy currents.
III.Analysis Results
The calculated flux and eddy current distributions are shown in Fig. 2. The skin effect of flux density can be observed in the flux distribution. To show the micro eddy currents clearly, the eddy current calculated by using the proposed method is subtracted with the classical one, and the obtained micro eddy currents are shown in Fig. 2 (b)(ii). We can see that micro eddy current around the domain walls are generated.
References
1 G. Bertotti, “Hysteresis in Magnetism: For physicists, Materials scientists, and Engineers”, Academic Press, San Diego, (1998).
2 A. J. Moses, “Energy efficient electrical steels: magnetic performance prediction and optimization”, Scripta Materialia, vol. 67 no. 6, pp. 560–565, 2012.
3 J. E. L. Bishop, “Enhanced eddy current loss due to domain displacement”, Journal of Magnetism and Magnetic Materials, vol. 49, no. 3, pp. 241-249, 1985.
4 R. H. Pry and C. P. Bean, “Calculation of the energy loss in magnetic sheet materials using a domain model,” Journal of Applied Physics, vol. 29, no. 3, pp. 532-533, 1958.
5 Y. Gao, Y. Matsuo, and K. Muramatsu, “Investigation on simple numeric modeling of anomalous eddy current loss in steel plate using modified conductivity,” IEEE Trans. on Magn., vol. 48, no. 2, pp, 635-638, 2012.

Fig. 1. Analysis model (D: sheet thickness).

Fig. 2. Flux and eddy current distributions.