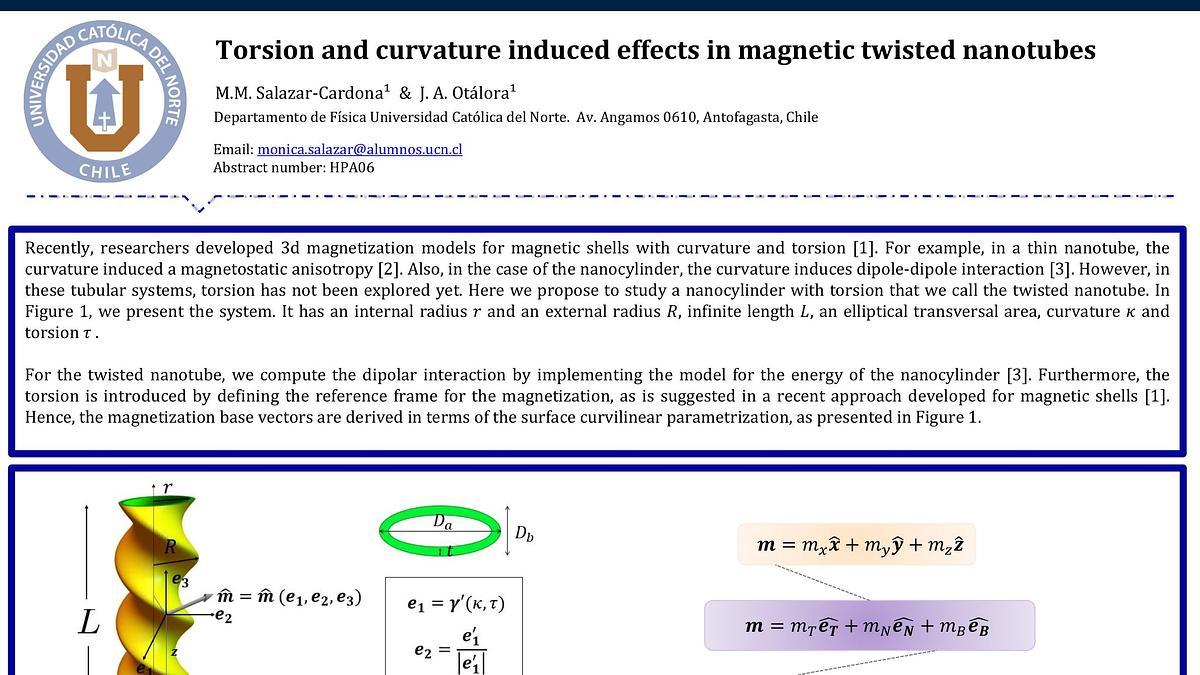
Recently, researchers developed 3d magnetization models for magnetic shells with curvature and torsion 1. For example, in a thin nanotube, the curvature induced a magnetostatic anisotropy 2. Also, in the case of the nanocylinder, the curvature induces dipole-dipole interaction 3. However, in these tubular systems, torsion has not been explored yet. Here we propose to study a nanocylinder with torsion that we call the twisted nanotube. In Figure 1, we present the system. It has an internal radius r, an external radius R, infinite length L, an elliptical transversal area, curvature κ and torsion τ.
Figure 1. The magnetization vector in terms of the base vectors (e₁ e₂, e₃). The parametrization of a 2D tubular surface γ, embedded in a 3D space defines these vectors.
For the twisted nanotube, we compute the dipolar interaction by implementing the model for the energy of the nanocylinder 3. Furthermore, the torsion is introduced by defining the reference frame for the magnetization, as is suggested in a recent approach developed for magnetic shells 1. Hence, the magnetization base vectors are derived in terms of the surface curvilinear parametrization, as presented in Figure 1. With this methodology, we show that in the twisted nanotube, there are volumetric charges because of the torsion of the system. In equation (1), we present the expression for the volumetric charges, where the term Γ_d is the contribution of the torsion. Also, the developed model shows that the torsion does not induce surface charges. With this work, we expect to contribute to future developments of spintronic technology, for example, supporting a theoretical model for a magnonic transducer, like the one developed for the nanocylinder 4.
▽ ● M = M sinΘ( sinp/ρ - ∂_zΘ + Γ_d) (1)

References
1 Gaididei, Yuri, Volodymyr P. Kravchuk, and Denis D. Sheka, "Curvature effects in thin magnetic shells," Physical review letters vol. 112, no. 25, pp. 257203, 2014.
2 Landeros, Pedro, and Álvaro S. Núñez, "Domain wall motion on magnetic nanotubes," Journal of Applied Physics vol. 108, no. 3, pp. 033917, 2010.
3 Otálora, J. A., et al, "Breaking of chiral symmetry in vortex domain wall propagation in ferromagnetic nanotubes," Journal of magnetism and magnetic materials vol. 341, pp. 86-92, 2013.
4 Salazar-Cardona, Monica M., et al, "Nonreciprocity of spin waves in magnetic nanotubes with helical equilibrium magnetization," Applied Physics Letters vol. 118, no. 26, pp. 262411, 2021.

Figure 1. The magnetization vector in terms of the base vectors (e₁, e₂, e₃). The parametrization of a 2D tubular surface γ, embedded in a 3D space defines these vectors.