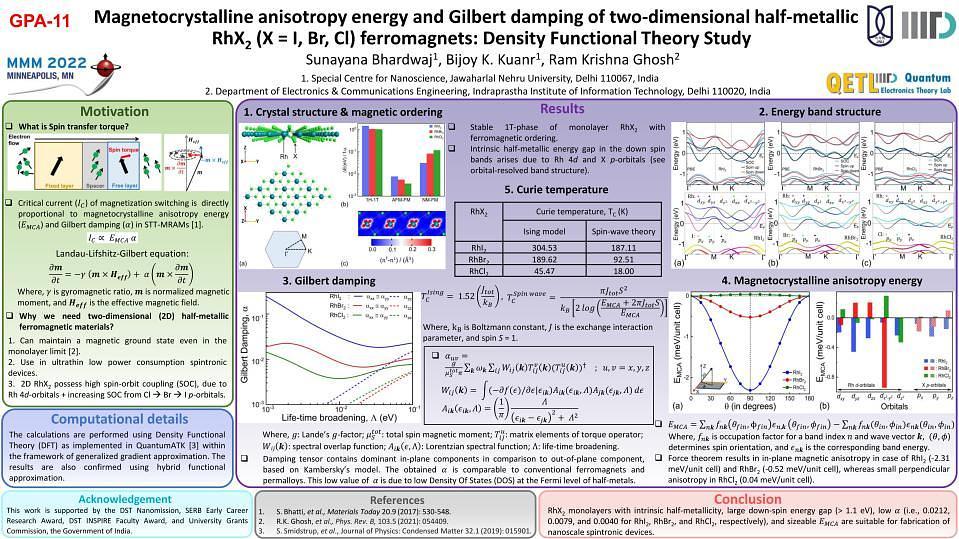
Two-dimensional (2D) intrinsic half-metallic ferromagnets (HMFs) are potential candidates for ultrathin spintronic device applications 1. The understanding of magnetocrystalline anisotropy energy (EMCA) and Gilbert damping (α) is required to determine their use in practical applications. In particular, the thermal stability and critical current of magnetization switching of spin-transfer-torque magnetic random-access memories highly depend on EMCA and α 2. Therefore, using density functional theory, we study the monolayer Rhodium dihalides family, RhX₂, where X= I, Br, Cl. First, we find that RhX₂ prefer 1T phase (octahedral symmetry) than the other possible 1H phase (trigonal prismatic symmetry). The ferromagnetic ordering is energetically favourable in RhX₂ monolayers. The Curie temperature of RhI₂, RhBr₂ and RhCl₂ crystals calculated using spin-wave theory (& Ising model) 3,4 is 182 (304) K, 92 (189) K and 17 (45) K respectively. We then calculate the spin-polarized electronic band structure which reveals wide intrinsic half-metallic gap (> 1.1 eV) in the down spin bands of RhX₂ monolayers. It results in 100% spin-polarization about the Fermi level and should prevent the spin-current leakage in nanoscale devices. The inclusion of spin-orbit coupling (SOC) does not affect the band’s profile much and the half-metallic character remains intact. Moreover, the metallic nature arises mainly from the hybridization of Rh 4d-orbitals and X p-orbitals which are present on the Fermi level. The EMCA and α which originate from SOC control the spin dynamics. We use force theorem 5 for EMCA calculation and the results show substantial in-plane magnetic anisotropy in RhI₂(-2.3 mJ/m²) and RhBr₂(-0.57 mJ/m²) whereas small perpendicular anisotropy in RhCl₂(0.04 mJ/m₂) 6. To calculate α we employ Kambersky's torque-torque correlation model 7 and it comes out relatively low (2.1×10^-2to 4.0×10^-3). This work highlights the importance of 2D RhX₂ HMFs in fabrication of future spintronic devices.
References
1 T. Song, X. Cai and M. W. Y. Tu, Science 360, 1214 (2018).
2 S. Ikeda, K. Miura and H. Yamamoto, Nat. Mater. 9, 721 (2010).
3 J. L. Lado and J. Fernández-Rossier, 2D Mater. 4, 035002 (2017).
4 J. M. Dixon, J. A. Tuszynski and E. J. Carpenter, Physica A 349, 487 (2005).
5 D. Li, C. Barreteau, M. R. Castell and F. Silly, Phys. Rev. B 90, 205409 (2014).
6 Ram Krishna Ghosh, Ashna Jose and Geetu Kumari, Phys. Rev. B 103, 054409 (2021).
7 K. Gilmore, Y. U. Idzerda and M. D. Stiles, Phys. Rev. Lett. 99, 027204 (2007).

Fig.1 a) Side view of 1T-RhX₂ monolayer and difference between spin charge densities about Rh and I atoms, b) Electronic band structure of RhI₂

Fig.2 a) E_MCA vs. θ, b) Gilbert damping tensors vs. life-time broadening.