Premium content
Access to this content requires a subscription. You must be a premium user to view this content.

poster
High precision 3D Reconstruction of Multiple Magnetic Targets Based on Center Weighting Method
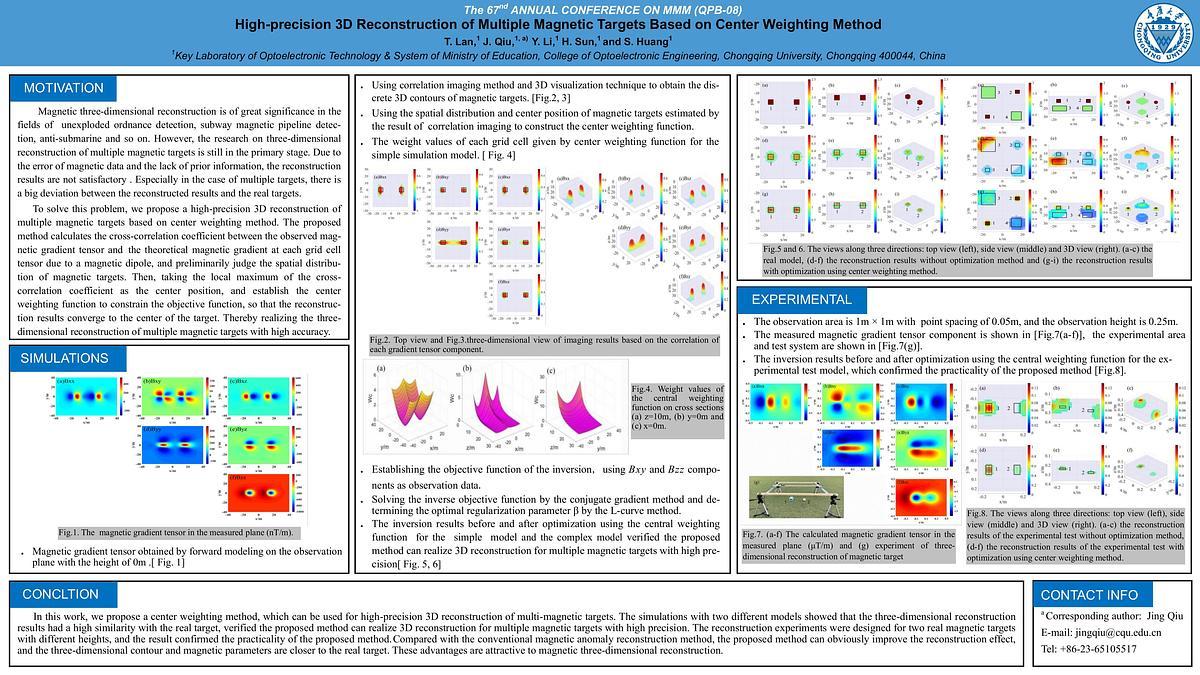
High-precision 3D reconstruction of multiple magnetic targets aims to reconstruct the three-dimensional shape, position and magnetic susceptibility of magnetic targets
based on discrete and noisy magnetic anomaly measurement data. It is widely used in anti-submarine, unexploded ordnance detection, subway magnetic pipeline detection and so on 1.
Currently, the research on three-dimensional reconstruction of multiple magnetic targets is still in the primary stage. Similar magnetic inversion technology is mainly studied in geological
exploration, including morphological inversion and physical inversion. For morphological inversion, it needs to know the magnetic susceptibility in advance, which leads to some
limitations in the reconstruction of unknown magnetic targets. For the physical inversion, because of the error of magnetic data and the lack of prior information, the effect of traditional
physical inversion method is not satisfactory 2. In order to solve this problem, this paper proposes a center weighting 3D imaging method based on normalized cross-correlation
coefficient. The method selects magnetic gradient tensor data as observation data, calculates its cross-correlation coefficient with each cuboid cells. This can reduce the interference caused
by magnetic measurement noise and errors of magnetic measurement parameters 3. And visualize the results to obtain the preliminary distribution of magnetic target (Figure 1). Then, the
spatial distribution and center position of the magnetic target are used as prior information to constrain the inversion equation. This can reduce the degree of freedom of the inversion
equation and get the optimal solution of the reconstruction result. Our simulation and experimental results show that the proposed method can realize 3D reconstruction of several simple
magnetic targets with a higher degree of fidelity (Figure 2).
References:
1 Y. Wang, I. I. Kolotov, D. V. Lukyanenko et al, Computational Mathematics and Mathematical Physics, Vol. 60, p. 1000–1007 (2020)
2 G. Yin, L. Zhang, Journal of Magnetism and Magnetic Materials, Vol. 482, p. 229–238 (2019)
3 Y. Li, J. Song, H. Xia et al, Journal of Applied Physics, Vol. 127,104701 (2020)



