Premium content
Access to this content requires a subscription. You must be a premium user to view this content.

poster
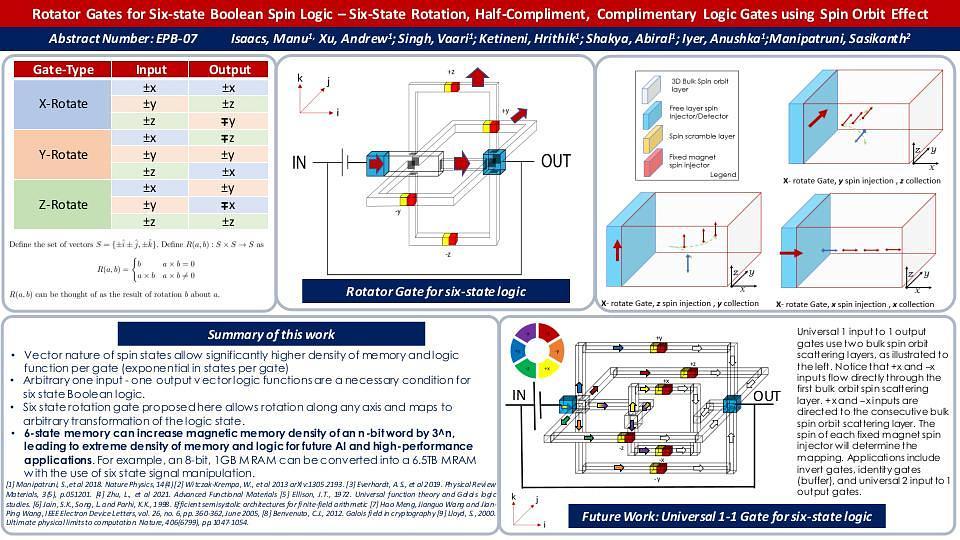
Rotator Gates for Six state Boolean Spin Logic
Spin state of currents and magnets offers a unique ability to store, transport and process information in vector boolean states leading to extreme density of memory and logic 1. We propose and demonstrate basic vector logic manipulation with six state magnetic and spin currents utilizing spin orbit effect in a reservoir gate. Recently investigated bulk spin orbit coupling (SOC) effects 2,3,4 hold a special solution to this complex logic problem due to the vector phenemenology of spin orbit interaction. We propose three critical gates for GF-n state logic 5, 6, known as inversion gate, and rotation gates (rotation around X axis, Y-axis and Z-axis) using the combination of spin torque switching and spin orbit switching. 6-state memory can increase magnetic memory density of an n-bit word by 3^n, leading to extreme density of memory and logic for future AI and high-performance applications. For example, an 8-bit, 1GB MRAM can be converted into a 6.5TB MRAM with the use of six state signal manipulation. When utilised the complete informaton density of six-state logic can extend the Moore's law for many generations by improving coding 7 and information density 8,9 without needing extreme lithography and materials scaling.
References
1 Manipatruni, S.,et al 2018. Beyond CMOS computing with spin and polarization. Nature Physics, 14(4), pp.338-343.
2 Witczak-Krempa, W., et al 2013. Correlated quantum phenomena in the strong spin-orbit regime. arXiv preprint arXiv:1305.2193.
Vancouver
3 Everhardt, A.S., et al 2019. Tunable charge to spin conversion in strontium iridate thin films. Physical Review Materials, 3(5), p.051201.
4 Zhu, L., et al 2021. Unveiling the Mechanism of Bulk Spin Orbit Torques within Chemically Disordered FePt Single Layers. Advanced Functional Materials, 31(36), p.2103898.
5 Ellison, J.T., 1972. Universal function theory and Galois logic studies. SPERRY RAND CORP ST PAUL MN UNIVAC DEFENSE SYSTEMS DIV. AD0740849.pdf (dtic.mil)
6 Jain, S.K., Song, L. and Parhi, K.K., 1998. Efficient semisystolic architectures for finite-field arithmetic. IEEE Transactions on Very Large Scale Integration (VLSI) Systems, 6(1), pp.101-113.
7 Benvenuto, C.J., 2012. Galois field in cryptography. University of Washington, 1(1), pp.1-11.
8 Lloyd, S., 2000. Ultimate physical limits to computation. Nature, 406(6799), pp.1047-1054.
9 Krauss, L.M. et al 2004. Universal limits on computation. arXiv preprint astro-ph/0404510.